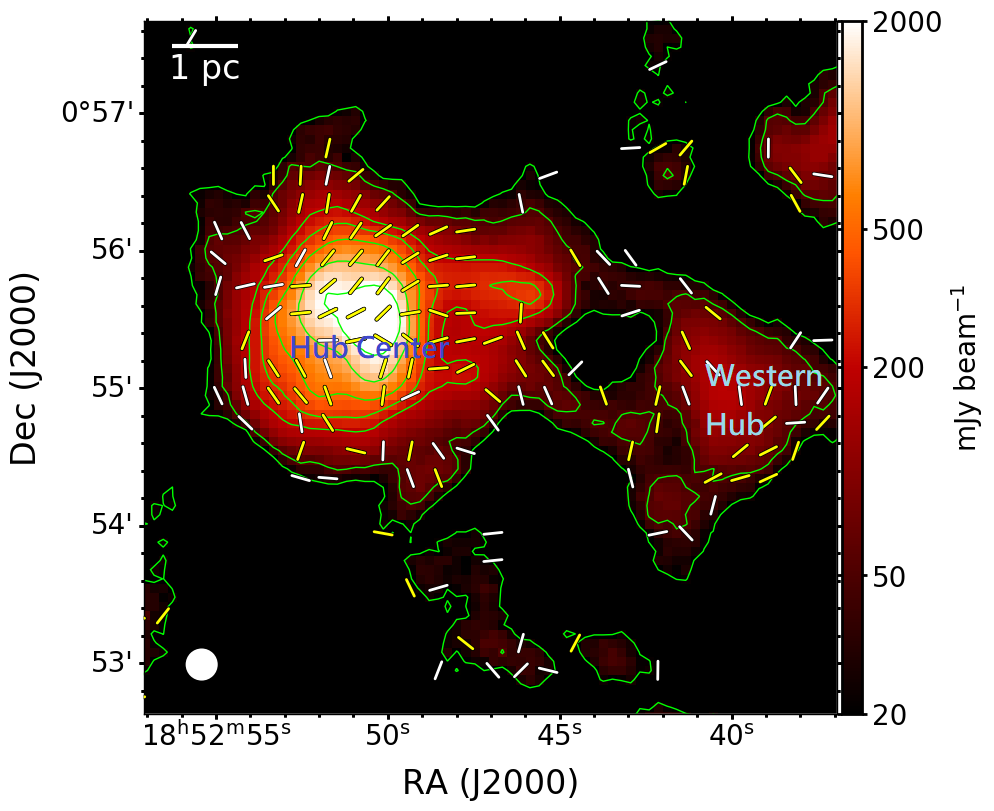
Figure 1. B-field orientations (segments) sampled on a 12” grid overlaid on 850 μm dust continuum (color and contours), sampled on a 4” grid, of the G33.92+0.11 region. The segments are rotated by 90° to represent magnetic field orientations. The yellow and white segments display the larger than 3 and 2–3 polarization detections. The green contours show the total intensity at 20, 50, 200, 300, 500, 1000, and 2000 mJy beam-1.
Interstellar filaments are ubiquitous in molecular clouds, and they are a key intermediate stage toward the formation of stars. Previous observations found that many stars commonly form within clustered environments associated with hub–filament systems (HFSs), where they are formed in a dense hub with numerous radial filaments extending from the central hub (Myers 2009, ApJ, 700:1609). Understanding how HFSs form is a topic of considerable interest since HFSs are the possible transition stage connecting the evolution of filamentary clouds and the formation of protoclusters.
G33.92+0.11 is such an HFS, where two massive protoclusters have been discovered by ALMA within the central 0.6 pc area of a dense hub associated with several few pc- length converging filaments (Liu et al. 2019, ApJ, 871:185). Since the size of the entire HFS is well beyond the maximum recoverable scale of interferometers, JCMT observations are essential to investigate the large-scale environments where the massive HFS could form.
We performed polarization observations using JCMT POL-2 to probe the magnetic field morphology in this 5-pc HFS. It is widely known that this polarized continuum emission originates from the dichoric alignment of interstellar dust grains along magnetic field lines in the interstellar medium through the Radiative Alignment Torques (RATs), and so the observed polarization orientation traces the plane-of-sky magnetic field morphology (Andersson et al. 2015 ARA&A, 53:501).
The magnetic field structure inferred from our POL-2 observations reveals a converging pattern pointing toward the hub center (Figure 1), apparently similar to the converging filamentary structures identified from the dust continuum map shown in the top panel of Figure 2, implying that the evolution of the converging filaments is coupled with magnetic fields.
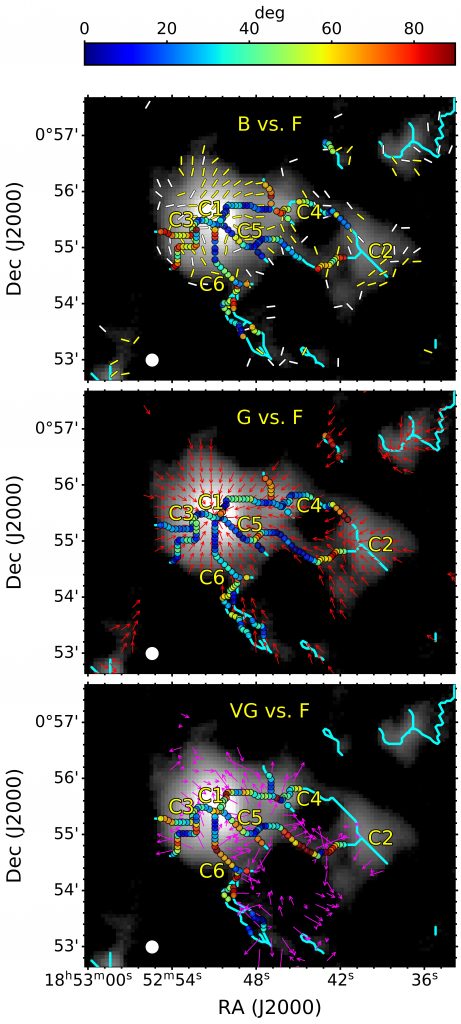
Figure 2: Differential orientation maps for filament vs. magnetic field, local gravity, and local velocity gradient (from top to bottom), overlaid on the 850 μm intensity. The cyan lines are the identified filaments. The yellow and white segments represent the magnetic field orientations, the red arrows are the projected local gravity, and the magenta arrows show the local velocity gradients. Filled color- coded circles (color wedge) are the pairwise differential orientations.
In order to evaluate the relative importance among gravity, turbulence, and magnetic fields, we used the modified Davis-Chandrasekhar-Fermi technique to estimate the energy scale of these physical parameters (Houde et al. 2009, ApJ, 706:1504). By combining our POL-2 polarization data and the velocity information extracted from the IRAM 30-m C18O (2-1) data, the obtained ratio of kinematic to gravitational energy is 0.10–0.20, and the ratio of magnetic to gravitational energy is 0.05–0.10. Hence, the global gravitational energy dominates the kinematic and magnetic energy and appears to be the major driving factor in the evolution of these filaments on a global scale.
The Davis-Chandrasekhar-Fermi technique only describes the averaged global properties over the entire system. It is important to note that many physical parameters, such as densities however, the physical parameters, including densities, gas velocities, magnetic field strengths, etc., are far from being homogeneous but actually vary by orders of magnitudes in such a dynamical HFS. Therefore, an analysis merely focusing on the global aspect might lack information on spatial variations which is essential for us to understand how the physical condition evolves from the ambient material to the converging filaments, and to the central hub. Hence, we additionally developed an approach aiming at studying the detailed local interplay between the spatial properties of the filamentary structures, the magnetic fields, the local gravitational force, and the local velocity gradient.
As a first step to investigate the spatial properties, we performed an all-pairwise comparison of the relative orientation among filaments, magnetic fields, local gravitational force, and local velocity gradient on the dust continuum map (Figure 2). This comparison reveals systematic changes of these relative orientations from diffuse extending filaments toward the densest hub center. With statistics based on the Kolmogorov-Smirnov test, we conclude that the filaments tend to align with the magnetic field and local gravity in the dense hub. In the low-density areas, we find that the local velocity gradients tend to be perpendicular to both the magnetic field and local gravity, although the filaments still tend to align with local gravity.
Combining local and global aspects, we propose a scenario where G33.92+0.11 is a multiscale gravitationally collapsing cloud with relatively weak turbulence and magnetic field. The ambient gas in the diffuse environment is accreted onto the filaments, while the filaments drag the magnetic field lines and flow toward the gravitational center (illustrated in Figure 3). Due to the resolution limitation, the observed local velocity gradients mainly trace the gas accumulation from the surrounding to the filaments, especially in low-density areas, and are thus perpendicular to the filaments. The observed magnetic field is stretched by the accretion flows, especially in high-density areas, and is therefore aligned with filaments and gravity.
One challenge of this scenario is how these converging filaments remain stable without fragmenting into numerous cores before reaching the hub center. To answer this question, we estimate the variation of critical linear density along these filaments considering the support from both thermal, non-thermal, and magnetic support. We find that the non-thermal kinematic energy within these filaments, traced by the local velocity dispersion, is significantly increasing with the local density. In return, this can stabilize filaments from self-fragmenting until reaching the central hub. This mechanism might also explain how a massive star/protocluster can accumulate a significant amount of mass from the large-scale environment.
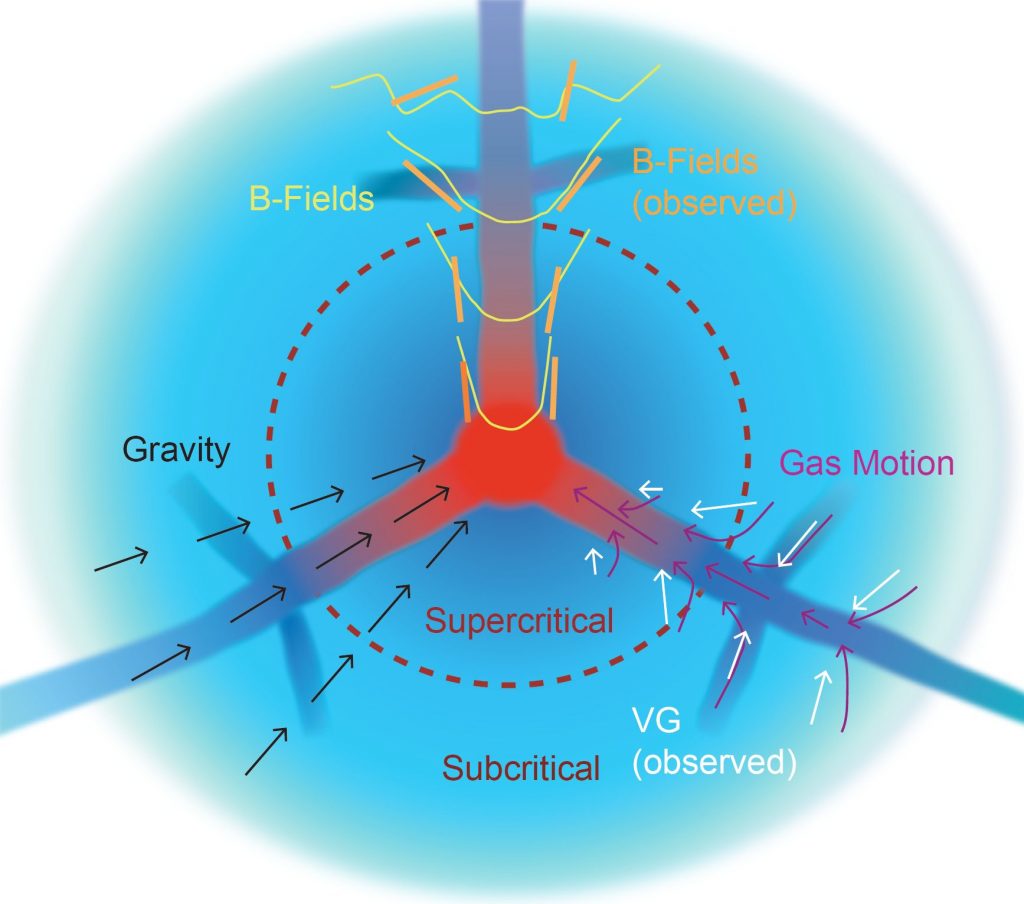
Figure 3: Cartoon illustrating observed features. The black arrows represent the directions of local gravity. The yellow curve shows a model-compatible magnetic field morphology, with the orange segments displaying the observed field segments with a spatial resolution (~0.5 pc) comparable to the filament widths (0.5–1 pc). The magenta arrows illustrate the directions of gas motion, with the white arrows depicting the observed velocity gradients at the resolved 0.5 pc scale. The background color displays local density. An outer subcritical and inner supercritical zone is indicated.
This research was published in The Astrophysical Journal at: https://iopscience.iop.org/article/10.3847/1538-4357/abc74e
Authors: Jia-Wei Wang1,2, Patrick M. Koch1, Roberto Galván-Madrid3, Shih-Ping Lai2, Hauyu Baobab Liu1, Sheng-Jun Lin2, and Kate Pattle2,4
Edited by Steve Mairs
Author Affiliations:
1 Academia Sinica Institute of Astronomy and Astrophysics, No. 1, Section 4, Roosevelt Road, Taipei 10617, Taiwan
2 Institute of Astronomy and Department of Physics, National Tsing Hua University, Hsinchu 30013, Taiwan; jwwang@asiaa.sinica.edu.tw
3 Instituto de Radioastronomía y Astrofísica, Universidad Nacional Autónoma de México, Apdo. Postal 3-72 (Xangari), 58089 Morelia, Michoacán, Mexico
4 Centre for Astronomy, School of Physics, National University of Ireland Galway, University Road, Galway, Ireland



